|
Kinetics Your feedback on these self-help problems is appreciated. Click here to send an e-mail. For on-line tutorial help with kinetics, there are four modules available in the Kinetics section of chemcal. |
Shortcut to Questions |
|||||||||
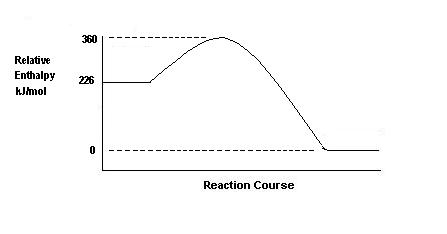
(a)
What is the meaning of EA on the diagram? 
(b)
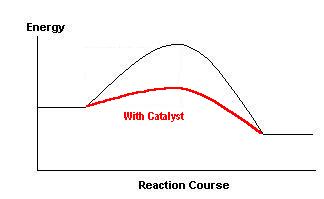
Indicate on the diagram the effect of the presence of a catalyst. |
||||||||||
| 2 |
Given below is a potential energy diagram for the reaction CO(g) + NO2(g) → CO2(g) + NO(g)
(a)
What is the activation energy for the reaction? |
|||||||||
| 3 |
For the reaction 2A + B + C → 3D + E the rate of formation of E is found to double if [A] is doubled, keeping [B] and [C] constant, and is found to double if [B] is doubled keeping [A] and [C] constant. The rate is unaffected by changes in [C]. Write the rate law for the reaction. |
|||||||||
| 4 |
For the reaction 2NO(g) + O2(g) → 2NO2(g) state the relationship between the rate of consumption of NO, the rate of consumption of O2, and the rate of production of NO2. |
|||||||||
| 5 |
The reaction between nitrite ion and iodide ion in acidic solution can be represented by the equation: 2NO2- + 2I- + 4H+ → I2 + 2NO + 2H2O The experimental rate
law for this reaction is (a)
[H+] and [I-] were kept constant but [NO2-]
were doubled? |
|||||||||
| 6 |
The rate of the reaction H3O+ + NH3 → NH4+
+ H2O was studied in 1960 and the rate expression
shown to be - R = k[H3O+][NH3] where
k = 4.3 x 1010 litre mole-1 second-1 at 298 K. Calculate the rate
of this acid-base reaction the instant 0.10 M ammonia (400 cm3)
is mixed with 0.050 M nitric acid (200 cm3). |
|||||||||
| 7 | Benzene diazonium chloride is found
to undergo hydrolysis as follows: At
298 K, the initial rate of hydrolysis was found to increase with
the initial concentration of benzene diazonium chloride as follows:
(a)
Use these results to determine the value of the reaction order,
n, in the rate equation:
Rate of formation of phenol = rate constant x [C6H5N2Cl]n (b)
Hence calculate the rate constant, k, in the rate equation above. (c)
The rate of the reaction can be expressed as the derivative d[C6H5OH] Choose
an alternative derivative expression for the rate, and give the
relationship between the two differentials. |
|||||||||
| 8 |
Dinitrogen
pentoxide decomposes in the solvent carbon tetrachloride according
to the following stoichiometric equation 2N2O5 → 4NO2 + O2 The
following data concerns this decomposition at 303 K.
(a)
Write the rate equation for the decomposition. (b)
Give the reaction order for the decomposition. (c)
Calculate the rate constant for the decomposition reaction at 303 K. |
|||||||||
| Answers to Kinetics Questions | ||||||||||
| (a)
EA is
the activation energy for the forward reaction. This is the minimum
energy that the colliding reactant molecules must possess before their
collisions can lead to the formation of product molecules.
(b)
The effect of the catalyst is to lower the height of the "hump".
By offering a new route the presence of the catalyst lowers EA
(for both forward and backward reactions). |
||||||||||
| 2 |
(a) From the graph, EA for the forward reaction = (360 - 226) = 134 kilojoule mole-1
= 360 kilojoule mole-1
(d)
At higher temperatures the proportion of molecules with the necessary
activation energy is increased. Rate of forward (and backward) reaction
is thus increased. |
|||||||||
| 3 |
Note that unlike equilibrium
constants, a rate law expression cannot be deduced from the stoichiometric
equation. Instead it requires a series of separate experiments to
deduce in turn the effect that the concentration of each reactant
has on the overall rate of the reaction. In a series of experiments,
only one reactant's concentration is altered in each experiment
while the concentrations of all the other reactants are held unchanged.
By these means, the effect of changing the concentration of each
reactant in turn on the rate of the overall reaction can be determined. |
|||||||||
| 4 |
In this question, the rate law is not requested and in fact the data needed to deduce it is not given. Here the simple stoichiometric relationship between the various reactants and products is all that is required to establish their relative rates of consumption or formation. In general terms, suppose that 1 molecule of a reactant A uses 2 molecules of another reactant B to form 1 molecule of product C, then the rate of consumption of B is twice that of A and that the rate of formation of C is identical to the rate of consumption of A.
|
|||||||||
| 5 | (a)
If only the [NO2-] is altered and is doubled,
then from the rate law, this would cause the rate to double as rate
∝
[NO2-]1. (b)
If only the [H+] is altered and is doubled, then from
the rate law, this would cause the rate to increase by a factor
of 4 as rate ∝ [H+]2. (d) If all concentrations were doubled, then the doubling
of [NO2-] would double the rate and doubling
the [I-] would double the rate again (now increased by
a factor of 4) and doubling the [H+] would increase the
rate by a further factor of 4 to give a total increase in rate of
16 times the original rate. |
|||||||||
| 6 |
The rate law is
rate = 4.3 x 1010 [H3O+][NH3]. Calculate the instantaneous
[H3O+] and [NH3] which have been
diluted upon mixing as follows: Total volume.=
600 mL. For dilutions,
the expression M1V1 = M2V2
is valid. so [H3O+]
= 1.67 x 10-2 M and for the diluted
[NH3], 0.10 x 400 = M2 x 600 so [NH3]
= 6.66 x 10-2 M Substituting these
values for [H3O+] and [NH3] in
the rate law Rate = 4.3 x 1010
[H3O+][NH3] = 4.3 x 1010 (1.67 x 10-2)(6.66 x 10-2) = 4.8 moles litre-1 second-1 (4.8 M s-1) |
|||||||||
| 7 | (a) By inspection of the data, doubling
the [C6H5N2Cl] causes the rate to
increase from 8.70 x 10-7 M s-1 to 1.74 x 10-6
M s-1, i.e. to double. ∴
rate is directly proportional to the [C6H5N2Cl],
so n = 1. (b) Substitute either set of data in the rate equation. 8.70 x 10-7 = k x 0.0200 ∴ k = 4.35 x 10-5
s-1. (c) See answer to Question
4 above for a discussion of the background to this part. From
the stoichiometry of the equation, each mole of C6H5OH
produced corresponds to the consumption of one mole of C6H5N2Cl.
Hence an alternative differential expression for the rate would
be -d
[C6H5N2Cl] /dt (The - sign indicates that species is being consumed) The
relation between them is d [C6H5OH] /dt = -d [C6H5N2Cl]
/dt
|
|||||||||
| 8 | (a)
By inspection of the data, doubling the concentration
of N2O5 causes the rate to double, so the rate
law is rate = k[N2O5]1. (b) As n = 1, reaction order = 1. (c) To evaluate k, substitute any of the sets of
data. 0.10
= k x 0.340 ∴ k = 2.9 x 10-1 hr-1. |
|||||||||