Electrolysis

Electrolysis Your feedback on these self-help problems is appreciated. Click here to send an e-mail.		Shortcut to Questions Q: 1 2 3 4 5 6 7 8
1	How many (a) faradays, (b) coulombs are required to deposit 0.587 g of Ni from a solution of Ni²⁺ ?
2	From a dilute solution of potassium tricyanocuprate(I), K₂[Cu(CN)₃], a uniform current deposits 0.586 g of copper in 108 minutes. What volume of hydrogen measured over water at 296 K and 746.0 mmHg would the same current liberate from dilute sulfuric acid in 81.0 minutes? (The equilibrium vapour pressure of water at 296 K = 20.9 mmHg.)
3	In the electrolysis of 0.100 M copper(II) sulfate solution, copper is deposited. How long would it be necessary to pass a 0.100 ampere current in order to deposit all of the copper from 1.00 litre of this solution?
4	Three electrolysis cells are connected in series. They contain, respectively, solutions of copper(II) nitrate, silver nitrate, and chromium(III) sulfate. If 1.00 g of copper is electrochemically deposited in the first cell, calculate the mass of silver and chromium deposited in the other cells.
5	A constant current of 3.7 milliampere is passed through molten sodium chloride for 9.0 minutes. The sodium produced is allowed to react with water (500 mL). What is the pH of the resulting solution?
6	A constant current of 0.126 ampere was passed through a dilute sulfuric acid solution for 149 minutes. The hydrogen produced was collected over mercury and the pressure adjusted to that of the surroundings (751 mmHg). The room temperature was 291 K. What was the volume of hydrogen produced?
7	One of the experimental ways of determining the Avogadro constant is by electrolysis. A current of 1.00 ampere is passed for 1.00 hour through a solution of potassium iodide. The weight of iodine liberated at the anode is 4.7135 g and there is no other anodic product. If the charge on the electron is 1.602 x 10^-19 coulomb, find from this set of data the Avogadro constant, N_A, correct to three significant figures. The atomic weight of iodine is 126.90.
8	Two electrolytic cells each containing two platinum electrodes were connected in series and a current of 0.500 ampere was passed for 16 minutes and 5 seconds. Cell 1 contained 0.100 M AgNO₃ and cell 2 contained 0.100 M CuSO₄. Calculate the following: (a) The change in mass of the platinum cathode in cell 1. (b) The volume of gas at 1.00 atmosphere and 273 K liberated at the platinum anode in cell 1. (c) The volume of gas at 1.00 atmosphere and 273 K liberated at the platinum anode in cell 2. (d) The change in mass of the platinum cathode in cell 2.
Electrolysis (Answers)
1	The reaction equation is Ni²⁺ + 2e^- → Ni From the stoichiometry of this equation, one mole of Ni produced requires the passage of two moles of electrons in the electrolysis. Mass of nickel produced = 0.587 g ∴ moles of Ni = mass/atomic wt = 0.587/58.71 = 1.00 x 10^-2 mol and moles of electrons required = 2 x 1.00 x 10^-2 = 2.00 x 10^-2 mol. One faraday of electrical charge (F) is one mole of electrons (i.e. 6.02 x 10²³ electrons - note that only the Avogadro number definition can apply to moles of electrons). ∴ number of faradays required = 2.00 x 10^-2 F One coulomb of charge (C) is the total charge involved when a current of one amp flows for one second. i.e. 1 amp = 1 coulomb flowing for 1 second. By experiment, one faraday is found to contain 96487 C per mole of electrons, the Faraday constant, F. ∴ coulombs in 2.00 x 10^-2 faradays = 96487 x 2.00 x 10^-2 = 1.93 x 10³ C
2	The first task in this question is to calculate from the amount of copper released, the current used in the electrolysis reactions. Then in the second part, knowing the current and the time it flows, the moles of electrons supplied to the H⁺ ions can be deduced. Then the moles of H₂ released and its volume under the stated conditions can be obtained. From the first electrolysis, deduce the moles of electrons: The reaction equation is Cu⁺ + e^- → Cu from which, one mole of electrons releases one mole of Cu. Mass of copper = 0.586 g ∴ moles of Cu = 0.586 / 63.55 = 9.221 x 10^-3 mol and moles of electrons also = 9.221 x 10^-3 mol. Convert moles of electrons to coulombs: Q = F x moles of electrons = 96487 x 9.221 x 10^-3 C = 8.897 x 10² C From the coulombs of charge, calculate the current: By the definition of the coulomb (see Q 1 answer), charge in coulombs = current in amps x time in seconds or Q = I x t ∴ I = Q / t = 8.897 x 10² / (108 x 60.0) =0.1373 amps Note that time must be expressed in seconds as required by the definition of the coulomb. Calculate the moles of electrons this current provides in 81.0 minutes: From Q = I x t, in 81.0 minutes (= 81.0 x 60.0 s), Q = 0.1373 x 81.0 x 60.0 = 6.673 x 10² C. Converting coulombs to moles of electrons, moles of electrons = coulombs / F = 6.673 x 10²/ 96487 = 6.916 x 10^-3 mol. Calculate the moles of H₂ released: The reaction equation is 2H⁺ + 2e^- → H₂ from which, one mole of H₂ formed requires two moles of electrons. ∴ moles of H₂ = ½ x 6.916 x 10^-3 = 3.458 x 10^-3 mol Calculate the volume of hydrogen gas formed: From the ideal gas equation PV = nRT, ∴ V = nRT / P n = 3.458 x 10^-3 mol R = 8.314 J K^-1 mol^-1 T = 296 K P = (746.0 - 20.9) mmHg = 725.1 mmHg or (725.1 / 760.0) atm or (725.1 / 760.0) x 101.3 kPa = 96.65 kPa. (Note that the total pressure must be reduced by the equilibrium vapour pressure of the water over which the hydrogen is collected in order to obtain the true pressure of hydrogen in the container.) Substituting this data, V = (3.458 x 10^-3 x 8.314 x 296) / 96.65 = 8.80 x 10^-2 L
3	The reaction equation is Cu^²⁺ + 2e^- → Cu From the stoichiometry of this equation, one mole of Cu^²⁺ requires the passage of two moles of electrons in the electrolysis. Moles of Cu^²⁺ in 1.00 L of 0.100 M solution = 0.100 mol. ∴ moles of electrons required = 0.200 mol or 0.200 F of charge. Q = 96487 x F = 96487 x 0.200 coulombs = 19297 C. Q = I x t for Q in coulombs, I in amps and t in seconds I = 0.100 amps ∴ t = Q / I = 19297 / 0.100 = 1.93 x 105 seconds or 1.93 x 105 / (60.0 x 60.0) hours = 53.6 hours.
4	The reaction equations are Cu^²⁺ + 2e^- → Cu Ag⁺ + e^- → Ag Cr³⁺ + 3e^- → Cr From the stoichiometry of these equations, one mole of electrons would deposit ½ mole of Cu in the electrolysis. As the cells are in series, the same current flows through all three cells and one mole of electrons would produce one mole of Ag and 1/3 mole of Cr from their solutions respectively. The moles of electrons used = 2 x moles of Cu deposited. Moles of Cu deposited = 1.00 / 63.55 = 1.574 x 10^-2 mol, so moles of electrons passed = 2 x 1.574 x 10^-2 = 3.148 x 10^-2 mol. In the silver cell: Moles of Ag deposited = moles of electrons passed = 3.148 x 10^-2 mol Mass of silver deposited = 3.148 x 10^-2 x 107.87 g = 3.40 g In the chromium cell: Moles of Cr deposited = (1/3) x moles of electrons passed = (3.148 x 10^-2) / 3 = 1.049 x 10^-2 mol Mass of chromium deposited = 1.049 x 10^-2 x 52.00 g = 0.546 g
5	The reaction equation is Na+ + e^- → Na From the stoichiometry of this equation, one mole of Na deposited requires the passage of one mole of electrons in the electrolysis. The sodium deposited is then reacted with water according to the equation Na + H₂O → Na⁺ + OH^- + ½H₂ From this equation, one mole of OH^- ions in the solution is formed from one mole of Na which in turn was deposited by the passage of one mole of electrons. ∴ moles of OH^- = moles of electrons passed Moles of electrons passed = Q / F = I x t / F I = 3.7 ma = 3.7 x 10^-3 amps (note that the units of current must be amps) t = 9.0 minutes = 9.0 x 60.0 seconds ∴ moles of electrons = (3.7 x 10^-3 x 9.0 x 60.0) / 96487 mol = 2.07 x 10^-5 mol. The solution contains 2.07 x 10^-5 mol of OH^- ions in 500 mL of water. ∴ [OH^-] = (2.07 x 10^-5) / 0.500 M = 4.14 x 10^-5 M pOH = -log[OH^-] = 4.38 pH = 14 00 - pOH = 9.62
6	The reaction equation is 2H⁺ + 2e^- → H₂ From the stoichiometry of this equation, one mole of H₂ produced requires the passage of two moles of electrons in the electrolysis. Calculate the moles of electrons passed. Moles of electrons = I x t / F I = 0.126 amps t = 149 x 60 seconds = 8940 seconds ∴ moles of electrons = 0.126 x 8940 / 96487 = 1.167 x 10^-2 mol. Calculate the moles of H₂ produced. From the equation above, moles of H₂ = ½ x moles of electrons = (1.167 x 10^-2) / 2 = 5.84 x 10^-3 mol Calculate the volume of hydrogen produced. Use the ideal gas equation rewritten as V = nRT / P where n = 5.84 x 10^-3 mol R = 8.314 JK^-1mol^-1 T = 291 K P = 751 mmHg = 751 / 760 atm = (751 / 760) x 101.3 kPa = 100.1 kPa [Note that there is no correction needed for the vapour pressure of mercury as it is negligible] ∴ V = (5.84 x 10^-3 x 8.314 x 291) / 100.1 = 0.141 L
7	The reaction equation is 2I^- → I₂ + 2e^- From the stoichiometry of this equation, one mole of I₂ produced requires the passage of two moles of electrons in the electrolysis. Calculate the moles of I₂ formed and thus moles of electrons passed. Moles of I₂ = mass of I₂ / GFW of I₂ = 4.7135 / (2 x 126.90) = 1.8572 x 10^-2 mol ∴ moles of electrons = 2 x 1.8572 x 10^-2 = 3.7143 x 10^-2 mol Calculate the total charge passed and thus the number of electrons. Q = I x t I = 1.00 amp t = 1.00 hour = 1.00 x 60.00 x 60.00 = 3600 sec (note the units of time must be seconds) ∴ Q = 1.00 x 3600 = 3600 C Given that the charge on one electron = 1.602 x 10^-19 C, ∴ number of electrons passed = 3600 / (1.602 x 10^-19) = 2.247 x 10²² electrons Thus deduce the number of electrons in one mole of electrons. As 2.247 x 10²² electrons = 3.7143 x 10^-2 mol, then 1.00 mol = (2.247 x 10²²) / (3.7143 x 10^-2) electrons = 6.05 x 10²³ electrons. ∴ N_A = 6.05 x 10²³.
8	Terminology: 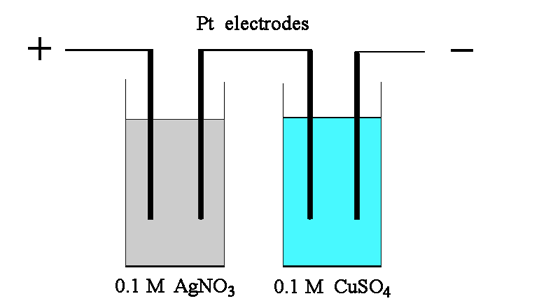Cathode is where reduction occurs and is ∴ the negative electrode Anode is where oxidation occurs and is ∴ the positive electrode. Cell diagram Set out the cell reactions for both cells. The reduction potentials for both Ag⁺ and Cu^²⁺ are larger than that for H⁺, so Ag and Cu will be deposited at the cathodes before H⁺ is reduced to H₂. The cell reactions are as follows. Cell 1 cathode: 2Ag⁺ + 2e^- → 2Ag Cell 1 anode: H₂O → 2H⁺ + ½O2(g) + 2e^- Cell 2 cathode: Cu^²⁺ + 2e^- → Cu Cell 2 anode: H₂O → 2H⁺ + ½O2(g) + 2e^- Calculate the moles of electrons passed. Moles of electrons = I x t / F I = 0.500 amp t = 16 min 5 sec = (16 x 60) + 5 sec = 965 sec ∴ moles of electrons = (0.500 x 965) / 96487 = 5.00 x 10^-3 mole Using the stoichiometry set out above, deduce the quantities of products. (a) Cell 1 cathode: In cell 1, moles of Ag formed = moles of electrons passed Mass of silver = moles x atomic wt = 5.00 x 10^-3 x 107.87 g = 0.539 g (increase in mass of electrode). (b) Cell 1 anode: In cell 1, moles of O₂ released = ¼ x moles of electrons passed = (5.00 x 10^-3) / 4 mole = 1.25 x 10^-3 mol Volume of oxygen released = nRT / P T = 273 K P = 1.00 atm = 101.3 kPa R = 8.314 ∴ V = (1.25 x 10^-3 x 8.314 x 273) / 101.3 = 0.0280 L or 28.0 mL (c) Cell 2 anode: In cell 2, the same volume of oxygen is liberated at the anode as the same current passes through both cells. (d) Cell 2 cathode: In cell 2, moles of Cu formed = ½ x moles of electrons passed = 2.50 x 10^-3 mol. Mass of copper = moles x atomic wt = 2.50 x 10^-3 x 63.55 g = 0.159 g (increase in mass of electrode).