where D_e is the bond dissociation energy, r_eq is the equilibrium bond length and a is a constant for a particular molecule. Using this function, the quantum mechanical energy levels (in cm^-1) are:

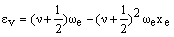
where w_e is the anharmonic oscillation frequency (see below) and x_e is the anharmonicity constant (which is small and positive). In the harmonic case, the vibrational levels are equally spaced. The second term in the anharmonic equation causes the levels to become more closely spaced as v increases. At high v values, the energy levels converge to the dissociation energy.

This equation can be rewritten in a form which can be compared with that for the harmonic oscillator:

harmonic		anharmonic

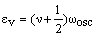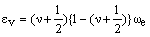which suggests that:

As x_e is positive, anharmonic oscillation frequency w_e is greater than w_osc and the anharmonic oscillator behaves like the harmonic oscillator but w_osc decreases with increasing v. For the hypothetical state with v = -1/2 corresponding to the bottom of the energy well when the vibrational energy would be zero, w_e = w_osc. The dissociation energy is related to the w_osc and x_e:

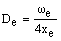
Input values into the calculator below for the force constant and atomic masses and press "calculate" to work out the energy levels.

The number of molecule in an energy level (N_v, compared to the number in the lowest level (N₀ = 0) is given by the Boltzmann distribution:

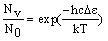
where De is the difference in energy between the level and the lowest possible level (v = 0). Input a temperature into the calculator and press "calculate" to work out the populations.

Once you have covered all of the available resources for vibrational spectroscopy, you should test your knowledge and understanding with the self test.

Hit the 'Graphical' button to see an interactive graphical version of this calculator.