Introduction to High Resolution Spectroscopy

For the hydrogen atom, the energy of the atomic orbitals depends only on the value of the principal quantum number, n. This is allowed to take positive integer values only: n = 1, 2, 3, 4, 5 ....

In the Bohr model, n is directly related to the radius, r, of the electron's orbit around the nucleus:

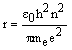
where ε₀ = 8.85x10^-12 J^-1C²m^-1 (permittivity of free space), m_e = 9.91x10^-31 kg (mass of an electron), e = 1.60x10^-19 C (charge of a proton) and h = 6.63x10^-34 Js (Planck's constant).

Task 1

Use the calculator below to calculate the Bohr radius for the H atom with n = 1, 2 and 3. Enter the value of the principal quantum number and press 'calculate radius '. Record the values in your laboratory notebook. (The answers are presented as powers of ten: for example 0.1e-01 means 0.1x10^-1)

Once you have covered all of the available resources for electronic spectroscopy, you should test your knowledge and understanding with the self test.

Bohr radius calculator
quantum number: n	Bohr radius: (m)	Bohr radius: (Å)

In the quantum mechanical atom, the Bohr radius should be interpreted as the most probable distance from the nucleus for the electron.
The energy of the electron increases as n increases because of the increased distance from the nucleus:

where R_H is the Rydberg constant for hydrogen. R_H = 1.097x10⁵ cm^-1 - read note on units for an explanation of units used in spectroscopy.

The energy is lowest (most negative) for n = 1. As n increases, the energy increases until the electron becomes ionized. At this point n is very large and the energy of the electron is zero.

As well as ionizing an electron, it is possible to excite an electron from a lower level to a higher level, requiring the absorption of energy. An excited electron will fall back down to a lower level, resulting in the emission of energy.
The energy absorbed or emitted can be calculated if the values of n for the lower and upper levels are known.
If the lower and upper levels have principal quantum number n₁ and n₂ respectively, their energies are:

lower level:

upper level:

and the energy absorbed or emitted, ΔE, is the difference between them:

For example, excitation of the electron from n₁ = 1 to n₂ = 2, requires:

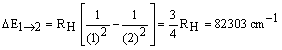
If this energy is provided with light, then it must be in the ultraviolet region of the spectrum.

Task 2

When an electric discharge is passed through H₂ molecules, they dissociate into excited H atoms. The H atoms emit light as the excited electron falls down to a lower level giving rise to a series of emissions, or a spectrum, at different frequencies.
The lines in the visible region are called the Balmer lines after their discoverer. The wavenumbers of the Balmer lines are shown in the table below.

wavenumber of light (cm^-1)	lower level: n₁	upper level: n₂


15241
20576
23045
24386
25196
25720
26080

Using the calculator below, complete the table by trying different values for n₁ and n₂. (Hint: all the lines correspond to a common lower level).

Enter the principal quantum number for the lower and upper level and press 'calculate'. (The answers are presented as powers of ten: for example 0.1e-01 means 0.1x10^-1)

H atom spectrum calculator
lower level: n₁	upper level: n₂
energy
wavelength (nm):	wavenumber (cm^-1):	frequency (Hz):

Task 3

The table below lists the wavenumbers for the Lyman and Paschen series observed in the ultraviolet and infrared respectively. As for the Balmer series, the lines in each series correspond to emission from excited levels to a common lower level. Using the calculator, obtain the lower level involved in both series.

Balmer	Lyman	Paschen


15241	82303	5335
20576	97543	7804
23045	102880	9145
24386	105350	9953
25196	106690	10478
25720	107500	10838
26080	108020	11096

lower level: n₁	lower level: n₁	lower level: n₁

Task 4

Use the calculator to work out the ionization energy for the H atom. This energy can be given as a wavelength (in nm), as a wavenumber (in cm^-1) or as a frequency (in Hz). These units, although not S.I. units, are commonly used in spectroscopy. If you want to obtain the answer in S.I. units, use the on-line converter to convert from these spectroscopic units.

Hint: ionization corresponds to raising the electron into a very high energy level from the lowest level. Try entering very large values for n₂. Does entering an even higher value change the answer?