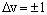
where an increase in v corresponds to absorption and a decrease in v corresponds to emission. The transition energy is the difference in energy between these two levels. If one level has vibrational quantum number v then absorption is to the level with quantum number (v+1). The energy of these levels is:

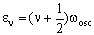
and

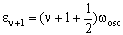
The energy difference is then:

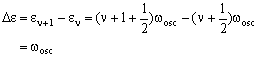
The transition energy is therefore independent of v and is just equal to the classical oscillation frequency.

At normal temperatures, the population of levels with v > 0 are very low so the spectrum is dominated by the peak corresponding to excitation from v = 0 to v = 1. The intensity of transitions starting from levels with v > 0 depends on the Boltzmann population of the lower level.

Input values into the calculator below for the force constant and atomic masses and press "calculate" to work out the energy levels and transition energies. Input a value for the temperature to work out the relative intensity of each transition.

Once you have covered all of the available resources for vibrational spectroscopy, you should test your knowledge and understanding with the self test.

Hit the 'Graphical' button to see the predicted spectrum and an interactive graphical version of this calculator.